Coordenadas de Rindler

En física relativista, la carta de coordenadas de Rindler es una importate y útil carta de coordenadas representando parte del espaciotiempo plano, también llamado el vacío de Minkowski. La carta de Rindler fue introducida por Wolfgang Rindler. El marco o sistema de coordenadas de Rindler describe un marco de referencia uniformemente acelerado en el espacio de Minkowski. En relatividad especial, una partícula uniformemente acelerada lleva un movimiento hiperbólico. Para cada partícula puede escogerse un marco de Rindler para el cual esta se encuentra en reposo.

Relación con la carta Cartesiana

Para obtener la carta de Rindler, se comienza con la carta cartesiana

$ds^{2}=-dT^{2}+dX^{2}+dY^{2}+dZ^{2},\;\;-\infty <T,X,Y,Z<\infty$

En la región $0<X<\infty ,\;-X<T<X$, que es a veces llamada la cuña de Rindler, se define la nueva carta usando la transformación de coordenadas

$t=\operatorname {arctanh} (T/X),\;x={\sqrt {X^{2}-T^{2}}},\;y=Y,\;z=Z$

La transformación inversa es

$T=x\,\sinh(t),\;X=x\,\cosh(t),\;Y=y,\;Z=z$

En la carta de Rindler, el elemento de linea de minkovsky se convierte en

$ds^{2}=-x^{2}dt^{2}+dx^{2}+dy^{2}+dz^{2},\;0<x<\infty ,-\infty <t,y,z<\infty$

Los observadores de Rindler

En la nueva carta, es natural tomar el campo de co-marco

$d\sigma ^{0}=-x\,dt,\;\;d\sigma ^{1}=dx,\;\;d\sigma ^{2}=dy,\;\;d\sigma ^{3}=dz$

el cual tiene el campo de marco dual

${\vec {e}}_{0}={\frac {1}{x}}\,\partial _{t},\;\;{\vec {e}}_{1}=\partial _{x},\;\;{\vec {e}}_{2}=\partial _{y},\;\;{\vec {e}}_{3}=\partial _{z}$

Esto define un marco local de Lorentz en el espacio tangente en cada evento (en la región cubierta por la carta de Rindle, a saber, la cuña de Rindler). Las curvas integrales del campo de vectores unitarios tipo tiempo ${\vec {e}}_{0}$ dan una congruencia tipo tiemo, consistente en las lineas de universo de una familia de observadores llamados los observadores de Rindler. En la carta de Rindler, estas líneas de universo aparecen como las líneas de la coordenada vertical $x=x_{0},\;y=y_{0},z=z_{0}$. Usando la transformación de coordenadas anterior, encontramos que estas corresponden a arcos de hipérbola en la carta cartesiana original.

Algunos observadores de Rindler representativos (arcos hiperbólicos azul marino) dibujados usando la carta cartesiana.

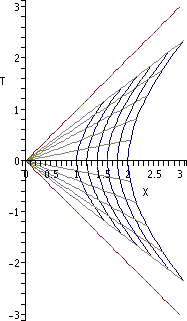
Como con cualquier congruencia tipo tiempo en cualquier variedad Lorentziana, esta congruencia tiene una descomposición cinemática (ver ecuación de Raychaudhuri). En este caso, la expansión y vorticidad de la congruencia de los observadores de Rindler se anula. La anulación del tensor de expansión implica que cada uno de los observadores mantiene una distancia constante con sus vecinos. La anulación del tensor de vorticidad implica que las líneas de universo de los observadores no están rotando unas sobre otras; este es un tipo de ausencia local de "arremolinamiento".

El vector aceleración de cada observador viene dado por la derivada covariante

$\nabla _{{\vec {e}}_{0}}{\vec {e}}_{0}={\frac {1}{x}}\,{\vec {e}}_{1}$

Esto es, cada observador de Rindler está acelerando en la dirección $\partial _{x}$. Individualmente hablando, cada observador está de hecho acelerando com magnitud constante en esta direción, por lo que sus líneas de universo son los análogos Lorentzianos de las cirunferencias, que son las curvas de curvatura constante en la geometría euclídea.

Debido a que los observadores de Rindler están libres de vorticidad, son también una hipersuperficie ortogonal. The hiperrebanadas espaciales ortogonales son $t=t_{0}$; estas aparecen como semiplanos horizontales en la carta de Rindler y como semiplanos atravesando $T=X=0$ en the carta cartesiana (ver la figura ariba). Haciendo $dt=0$ en el elemento de línea, vemos que estas tienen la geometría eclidiana ordinaria, $d\sigma ^{2}=dx^{2}+dy^{2}+dz^{2},\;0<x<\infty ,\;-\infty <y,z<\infty$. Así, las coordenadas espaciales en la carta de Rindler tienen una interpretación muy simple consistente con la afirmación de que los observadores de Rindler son mutumente estacionarios. Volveremos a esta propiedad de rigidez de los observadores de Rindler un poco más tarde en este artículo.

Una propiedad "paradójica"

Nótese que los observadores de Rindler con coordenada x más pequeña y constante. ¡están acelerando más fuerte para mantenerse al mismo nivel! Esto puede parecer sorprendente porque en física newtoniana, los observadores que mantienen distancia relativa constante deben compartir la misma aceleración. Pero en física relativista, vemos que el extremo trasero de una barra que está accelerada por alguna fuerza externa (paralela a su eje de simetría) debe acelerar un poco más fuerte que el extremo delantero, o si no eventualmente se romperá.

Este fenómeno es la base de una bien conocida "paradoja". En todo caso, es una simple consecuencia de la cinemática relativista. Una forma de ver esto es observar que la magnitud del vector aceleración es justamente la curvatura de la línea de universo correspondiente. Pero las líneas de universo de los observadores de Rindler son los análogos de una familia de circunferencias concéntricas en el plano euclídeo, por lo que estamos lidiando simplemente con el análogo lorentziano de un hecho familiar: al girar en una familia de circunferencias concéntricas, los círculos más pequeños deben doblarse más rápido (por unidad de arco) que los exteriores.

Observadores de Minkowski

Un observador de Minkowski representativo (curva hperbólica secante azul marino) dibujada usando la carta de Rindler. El horizonte de Rindler está mostrado en rojo.

Vale la pena introducir también un marco alternativo, dado en la carta de Minkowski por la elección natural

${\vec {f}}_{0}=\partial _{T},\;{\vec {f}}_{1}=\partial _{X},\;{\vec {f}}_{2}=\partial _{Y},\;{\vec {f}}_{3}=\partial _{Z}$

Transformando estos campos de vectores usando la transformación de coordenadas dada anteriormente, encontramos que en la carta de Rindler (en la cuña de Rinder) este marco se convierte en

${\vec {f}}_{0}={\frac {\cosh(t)}{x}}\,\partial _{t}-\sinh(t)\,\partial _{x}$

${\vec {f}}_{1}=-{\frac {\sinh(t)}{x}}\,\partial _{t}+\cosh(t)\,\partial _{x}$

${\vec {f}}_{2}=\partial _{y},\;{\vec {f}}_{3}=\partial _{z}$

Calculando la descomposición cinemática de la congruencia tipo tiempo definida por el campo de vectores unitarios tipo tiempo ${\vec {f}}_{0}$, encontramos que la expansión y la vorticidad de nuevo se anulan, y además el vector aceleración también se anula, $\nabla _{{\vec {f}}_{0}}{\vec {f}}_{0}=0$. En otras palabras, esta es una congruencia geodésica; los observadores correspondientes están en un estado de movimiento inercial. En la carta cartesiana original, estos observadores, a quienes vamos a llamar observadorse de Minkowski, están en reposo.

En la carta de Rindler, las líneas de universo de los observadores de Minkowski aparecen como curvas secantes hiperbólicas asintóticas al plane plano $x=0$. Especificamente, en coordenadas de Rindler, la línea de universo de un observador de Minkowski pasando a través del evento $t=t_{0},\;x=x_{0},\;y=y_{0},\;z=z_{0}$ es

$t=\operatorname {arctanh} (s/x_{0}),\;x={\sqrt {x_{0}^{2}-s^{2}}},\;y=y_{0},\;z=z_{0},\;-x_{0}<s<x_{0}$

donde $s$ es el tiempo propio de este observador de Minkowski. Nótese que sólo una pequeña parte de su historia es cubierta por la carta de Rindler. Esto muestra explicitamente por qué la carta de Rindler no es geodesicamente completa; geodésicas tipo tiempo salen fuera de la región cubierta por la carta en un tiempo propio finito. Por supuesto, sabemos que la carta de Rindler no puede ser geodésicamente completa,porque sólo cubre una parte de la carta cartesiana original, que es geodésicamente completa.

En el caso mostrado en la figura, $x_{0}=1$ y hemos dibujado (correctamente escalado) los conos de luz en $s=-{\frac {1}{2}},\;0,\;{\frac {1}{2}}$.

El horizonte de Rindler

La carta de coordenadas de Rindler tiene unaa singularidad de coordenadas en $x=0$, donde el tensor métrico (expresado en las coordenadas de Rindler) tiene un determinante nulo. Esto ocurre porque mientras $x\rightarrow 0$ la aceleración de los observadores de Rindler diverge. Como podemos ver por la figura que iulstra la cuña de Rindler, el locus $x=0$ en la carta de Rindler corresponde al locus $T^{2}=X^{2},\;X>0$ en la carta cartesiana, que consiste en dos semiplanos nullos, cada uno regido por una congruencia geodésica nula.

Por el momento, simplemente consideraremos el horizonte de Rindler como el contorno de las coordenadas de Rindler. Más tarde veremos que es de hecho análogo en algunos importantes aspectos al horizonte de eventos en un agujero negro.

Geodésicas

Las ecuaciones geodésicas en la carta de Rindler son fácilmente obtenidas de el lagrangiano; son

${\ddot {t}}+{\frac {2}{x}}\,{\dot {x}}\,{\dot {t}}=0,\;{\ddot {x}}+x\,{\dot {t}}^{2}=0,\;{\ddot {y}}=0,\;{\ddot {z}}=0$

Por supuesto, en la carta cartesiana original, las geodésicas aparecen como líneas rectas, por lo que podemos obtenerlas fácilmente en la carta de Rindler usando la transformación de coordenadas. Sin embargo, es instructivo obtenerlas y estudiarlas independentemente de la carta original, y haremos eso en esta sección.

Algunas geodésicas nulas representativas (arcos de hipérbola semicirculares negros) proyectados en la hiperrebanada espacial t=0 de los observadores de Rindler. El horizonte de Rindler está mostrado como un plano magenta.

De la primera, tercera y cuarta obtenemos inmediatamenet las integrales primeras

${\dot {t}}={\frac {E}{x^{2}}},\;\;{\dot {y}}=P,\;\;{\dot {z}}=Q$

Pero por el elemento de línea tenemos $\epsilon =-x^{2}\,{\dot {t}}^{2}+{\dot {x}}^{2}+{\dot {y}}^{2}+{\dot {z}}^{2}$ where $\epsilon =-1,\,0,\,1$ para las geodésicas tipo tiempo, nulas y tipo espacio, respectivamente. Esto da la cuarta primera integral, a saber

${\dot {x}}^{2}=\left(\epsilon +{\frac {E^{2}}{x^{2}}}\right)-P^{2}-Q^{2}$.

Esto basta para dar la completa solución de las ecuaciones geodésicas.

En el caso de geodésicas nulas, de ${\frac {E^{2}}{x^{2}}}-P^{2}-Q^{2}$ con $E$ diferente de cero, vemos que la coordenada x varía en el intervalo$0<x<{\frac {E}{\sqrt {P^{2}+Q^{2}}}}$.

La familia de siete parámetros completa que da cualquier geodésica nula a través de cualquier evento en la cuña de Rindler es

${\begin{matrix}t-t_{0}&=&\operatorname {arctanh} \left({\frac {s\,(P^{2}+Q^{2})-{\sqrt {E^{2}-(P^{2}+Q^{2})\,x_{0}^{2}}}}{E}}\right)\\&&+~\operatorname {arctanh} \left({\frac {\sqrt {E^{2}-(P^{2}+Q^{2})\,x_{0}^{2}}}{E}}\right)\end{matrix}}$

$x={\sqrt {x_{0}^{2}+2\,s\,{\sqrt {E^{2}-(P^{2}+Q^{2})\,x_{0}^{2}}}-s^{2}\,(P^{2}+Q^{2})}}$

$y-y_{0}=P\,s;\;\;z-z_{0}=Q\,s$

Dibujando los caminos de algunas geodésicas nulas representativas a través de un evento dado (esto es, proyectando a la hiperrebanada $t=0$), obtenemos un dibujo que se parece sospechosamente a la familia de todas las semicircunferencias que atraviesan un punto ortogonales al horizonte de Rindler (ver figura).

La métrica de Fermat

The fact that in the Rindler chart, the projections of null geodesics into any spatial hyperslice for the Rindler observers are simply semicircular arcs can be verified directly from the general solution just given, but there is a very simple way to see this. A static spacetime is one in which a vorticity-free timelike Killing vector field can be found. In this case, we have a uniquely defined family of (identical) spatial hyperslices orthogonal to the corresponding static observers (who need not be inertial observers). This allows us to define a new metric on any of these hyperslices which is conformally related to the original metric inherited from the spacetime, but with the property that geodesics in the new metric (note this is a Riemannian metric on a Riemannian three-manifold) are precisely the projections of the null geodesics of spacetime. This new metric is called the Fermat metric, and in a static spacetime endowed with a coordinate chart in which the line element has the form

$ds^{2}=g_{00}\,dt^{2}+g_{jk}\,dx^{j}\,dx^{k},\;\;1\leq j,\;k\leq 3$

the Fermat metric on $t=0$ is simply

$d\rho ^{2}={\frac {g_{jk}\,dx^{j}\,dx^{k}}{-g_{00}}}$

(where the metric coeffients are understood to be evaluated at $t=0$).

In the Rindler chart, the timelike translation $\partial _{t}$ is such a Killing vector field, so this is a static spacetime (not surprisingly, since Minkowski spacetime is of course trivially a static vacuum solution of the Einstein field equation). Therefore, we may immediately write down the Fermat metric for the Rindler observers:

$d\rho ^{2}={\frac {dx^{2}+dy^{2}+dz^{2}}{x^{2}}},\;\;0<x<\infty ,\;\;-\infty <y,z<\infty$

But this is the well-known line element of hyperbolic three-space H³ in the upper half space chart! This is closely analogous to the well known upper half plane chart for the hyperbolic plane H², which is familiar to generations of complex analysis students in connection with conformal mapping problems (and much more), and many mathematically minded readers already know that the geodesics of H² in the upper half plane model are simply semicircles (orthogonal to the circle at infinity represented by the real axis).

Symmetries

Since the Rindler chart is a coordinate chart for Minkowski spacetime, we expect to find ten linearly independent Killing vector fields. Indeed, in the Cartesian chart we can readily find ten linearly independent Killing vector fields, generating respectively one parameter subgroups of time translation, three spatials, three rotations and three boosts. Together these generate the (proper isochronous) Poincaré group, the symmetry group of Minkowski spacetime.

However, it is instructive to write down and solve the Killing vector equations directly. We obtain four familiar looking Killing vector fields

$\partial _{t},\;\;\partial _{y},\;\;\partial _{z},\;\;-z\,\partial _{y}+y\,\partial _{z}$

(time translation, spatial translations orthogonal to the direction of acceleration, and spatial rotation orthogonal to the direction of acceleration) plus six more:

$\exp(\pm t)\,\left({\frac {y}{x}}\,\partial _{t}\pm \left(y\,\partial _{x}-x\,\partial _{y}\right)\right)$

$\exp(\pm t)\,\left({\frac {z}{x}}\,\partial _{t}\pm \left(z\,\partial _{x}-x\,\partial _{z}\right)\right)$

$\exp(\pm t)\,\left({\frac {1}{x}}\,\partial _{t}\pm \partial _{x}\right)$

(where the signs are chosen consistently + or -). We leave it as an exercise to figure out how these are related to the standard generators; here we wish to point out that we must be able to obtain generators equivalent to $\partial _{T}$ in the Cartesian chart, yet the Rindler wedge is obviously not invariant under this translation. How can this be? The answer is that like anything defined by a system of partial differential equations on a smooth manifold, the Killing equation will in general have locally defined solutions, but these might not exist globally. That is, with suitable restrictions on the group parameter, a Killing flow can always be defined in a suitable local neighborhood, but the flow might not be well-defined globally. This has nothing to do with Lorentzian manifolds per se, since the same issue arises in the study of general smooth manifolds.

Notions of distance

One of the many valuable lessons to be learned from a study of the Rindler chart is that there are in fact several distinct (but reasonable) notions of distance which can be used by the Rindler observers.

Operational meaning of the radar distance between two Rindler observers (navy blue vertical lines). The Rindler horizon is shown at left (red vertical line). The world line of the radar pulse is also depicted, together with the (properly scaled) light cones at events A, B, C.

The first is the one we have tacitly employed above: the induced Riemannian metric on the spatial hyperslices $t=t_{0}$. We will call this the ruler distance since it corresponds to this induced Riemannian metric, but its operational meaning might not be immediately apparent.

From the standpoint of physical measurement, a more natural notion of distance between two world lines is the radar distance. This is computed by sending a null geodesic from the world line of our observer (event A) to the world line of some small object, whereupon it is reflected (event B) and returns to the observer (event C). The radar distance is then obtained by dividing the round trip travel time, as measured by an ideal clock carried by our observer.

(In Minkowski spacetime, fortunately, we can ignore the possibility of multiple null geodesic paths between two world lines, but in cosmological models and other applications things are not so simple! We should also caution against assuming that this notion of distance between two observers gives a notion which is symmetric under interchanging the observers!)

In particular, let us consider a pair of Rindler observers with coordinates $x=x_{0},\;y=0,\;z=0$ and $x=x_{0}+h,\;y=0,\;z=0$ respectively. (Note that the first of these, the trailing observer, is accelerating a bit harder, in order to keep up with the leading observer). Setting $dy=dz=0$ in the Rindler line element, we readily obtain the equation of null geodesics moving in the direction of acceleration:

$t-t_{0}=\log(x/x_{0})$

Therefore, the radar distance between these two observers is given by

$x_{0}\,\log \left(1+{\frac {h}{x_{0}}}\right)=h-{\frac {h^{2}}{2\,x_{0}}}+O\left(h^{3}\right)$

This is a bit smaller than the ruler distance, but for nearby observers the discrepancy is negligible.

A third possible notion of distance is this: our observer measures the angle subtended by a unit disk placed on some object (not a point object!), as it appears from his location. We call this the optical diameter distance. Because of the simple character of null geodesics in Minkowski spacetime, we can readily determine the optical distance between our pair of Rindler observers (aligned with the direction of acceleration). From a sketch it should be plausible that the optical diameter distance scales like $h+{\frac {1}{x_{0}}}+O\left(h^{3}\right)$. Therefore, in the case of a trailing observer estimating distance to a leading observer (the case $h>0$), the optical distance is a bit larger than the ruler distance, which is a bit larger than the radar distance. The reader should now take a moment to consider the case of a leading observer estimating distance to a trailing observer!

There are other notions of distance, but the main point is clear: while the values of these various notions will in general disagree for a given pair of Rindler observers, they all agree that every pair of Rindler observers maintains constant distance. The fact that very nearby Rindler observers are mutually stationary follows from the fact, noted above, that the expansion tensor of the Rindler congruence vanishes identically. However, we have shown here that in various senses, this rigidity property holds at larger scales. This is truly a remarkable rigidity property, given the well-known fact that in relativistic physics, no rod can be accelerated rigidly (and no disk can be spun up rigidly) --- at least, not without sustaining inhomogeneous stresses. The easiest way to see this is to observe that in Newtonian physics, if we "kick" a rigid body, all elements of matter in the body will immediately change their state of motion. This is of course incompatible with the relativistic principle that no information having any physical effect can be transmitted faster than the speed of light.

It follows that if a rod is accelerated by some external force applied anywhere along its length, the elements of matter in various different places in the rod cannot all feel the same magnitude of acceleration if the rod is not to extend without bound and ultimately break. In other words, an accelerated rod which does not break must sustain stresses which vary along its length. Furthermore, in any thought experiment with time varying forces, whether we "kick" an object or try to accelerate it gradually, we cannot avoid the problem of avoiding mechanical models which are inconsistent with relativistic kinematics (because distant parts of the body respond too quickly to an applied force).

Returning to the question of the operational significance of the ruler distance, we see that this should be the distance which our observers will obtain should they very slowly pass from hand to hand a small ruler which is repeatedly set end to end. But justifying this interpretation in detail would require some kind of material model.

See also

• Bell's spaceship paradox, for a sometimes controversial subject often studied using Rindler coordinates.
• Born coordinates, for another important coordinate system adapted to the motion of certain accelerated observers in Minkowski spacetime.
• Congruence (general relativity)
• Ehrenfest paradox, for a sometimes controversial subject often studied using Born coordinates.
• Frame fields in general relativity
• General relativity resources
• Raychaudhuri equation
• Unruh effect

References

Useful background:

• Boothby, William M. (1986). An Introduction to Differentiable Manifolds and Riemannian Geometry. New York: Academic Press. ISBN 0-12-116052-1.  See Chapter 4 for background concerning vector fields on smooth manifolds.

• Frankel, Theodore (1979). Gravitational Curvature: an Introduction to Einstein's Theory. San Francisco : W. H. Freeman. ISBN 0-7167-1062-5.  See Chapter 8 for a derivation of the Fermat metric.

Rindler coordinates:

• Misner, Charles; Thorne, Kip S. & Wheeler, John Archibald (1973). Gravitation. San Francisco: W. H. Freeman. ISBN 0-7167-0344-0.  See Section 6.6.

• Rindler, Wolfgang (2001). Relativity: Special, General and Cosmological. Oxford: Oxford University Press. ISBN 0-19-850836-0. 

Rindler horizon:

• Jacobson, Ted; and Parenti, Renaud (2003). «Horizon Entropy». Found. Phys. 33: 323-348. doi:10.1023/A:1023785123428.  eprint version

• Barceló, Carlos; Liberati, Stefano; and Visser, Matt. «Analogue Gravity». Living Reviews in Relativity. Consultado el 6 de mayo de 2006.